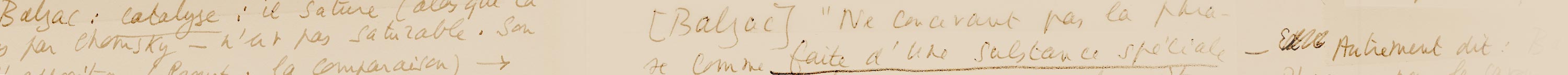• Tilman Sauer (Johannes Gutenberg Universität Mainz), How did Leibinz solve the catenary problem?

Abstract: The problem to find the exact shape of a heavy hanging chain of fixed length, the catenary line, is a famous problem in the early history of calculus. The linea catenaria, being a transcendental curve closely linked to the logarithmic and exponential curves, played a major role in Leibinz’s heuristics and his understanding of analysis. Leibniz was very proud of his solution of the catenary problem but never revealed publicly how he arrived at it. While it is known how Christiaan Huygens arrived at his solution of the catenary problem, Leibniz’s discovery path has remained largely unknown to date. In my presentation, I will present and discuss unpublished manuscripts by Leibniz that shed light on this question.

• David Waszek (ITEM, ÉNS-PSL), Analogies through notations. Leibniz’s analogies between powers and differences in light of his drafts

In the 1690s, Leibniz explored a number of analogies between powers and differentials. The best known is his analogy between the powers of a sum, (x+y)^n, and the higher differentials of a product, d^n(xy) (it is by reference to this analogy that the binomial-like formula giving the n-th derivative of a product of functions is often called “Leibniz’s formula” in textbooks today). However, as his correspondence and drafts testify, Leibniz’s research involved various other parallels, including: analogies between the development of fractions or roots into infinite series though the binomial formula, and the development of integrals into infinite series; attempts to clarify a “transcendantal” law of homogeneity for higher differentials that would parallel the usual law of homogeneity for algebraic terms; musings on fractional differentials; and parallels between the behaviour of algebraic and differential equations. The material that has been published so far (mostly Leibniz’s published articles and his correspondence with Johann Bernoulli) only gives us access to a biased and limited sample of Leibniz’s investigations. This talk explores Leibniz’s drafts on the topic, with a particular focus on his efforts to design notations and craft analogies despite the fact that the algebraic and differential domains often did not line up quite in the way he expected. I will be particularly interested in teasing out the specific values guiding Leibniz’s research practices, as well as in the role of notations in the trajectory of his research.